Yesterday I tutored a student taking Calculus AB and earlier that day I was teaching my Algebra 1 students how we can find the slope of a line given two points on the line and realized I was connecting a major theorem in calculus using the same logic I was teaching my Algebra 1 students.
In Algebra 1 we learn that the slope of the line is basically the ratio of the change in the y-coordinate to the change in the x-coordinate also known as (Rise over Run). To find this ratio we find the difference in our y's and divided it by the difference in our x's.
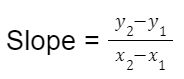
This concept will be important when trying to explain the Mean Value Theorem (MVT) once you get to Calculus. Here is the MVT in math language first then I'll try to explain it in everyday language for anyone who might not understant the language (Don't worry you're not alone).
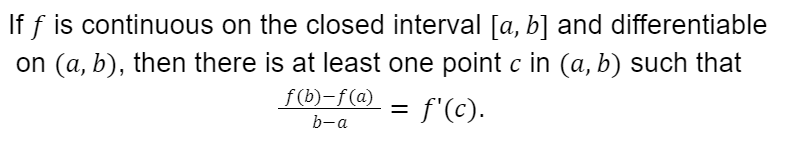
So the first thing we need to understand about the Mean Value Theorem is that is is a theorem that basically guarantee an x value c in the open interval (a,b) where the function is changing at the same rate as the average rate of change between the two endpoints of the interval [a,b] given two conditions.
The 2 Conditions in order for the MVT to apply:
1. First the function must be continuous in the close interval [a,b]. From the point (a,f(a)) to the point (b,f(b)), there must be no breaks in the function.
2. Second the function must be differentiable in the open interval (a,b) meaning there are no sharp turns in the graph of f(x).
Now the meaning:
If the two conditions apply, then at some point in the function the rate of change must be the same as the average rate of change between the two endpoints. The average rate of change between the endpoints is basically the slope of the line that would connect the two endpoints. Remember (difference in our y's over the difference in our x's from Algebra 1) Now that we are in Calculus we were able to find the instantanous rate of change at any given point on the function by finding the derivative f'(x) of the function f. So what the theorem tells us is that there will be at least 1 value c in between a and b such that the derivative of f at c in other words f'(c) will equal to the slope of the line that connected the two endpoints.
Future Engineers and Physicist: Pay close attention to the MVT as it is often used to analyze motion, such as determining when an object must have had a certain velocity given its average velocity over a period.:
Hope you enjoyed this blog and was helpful to you.
Don't forget to visit my website:
コメント